Numerical Simulations of Double-Diffusive Convection in Different Liquid Metals Under Magnetic Fields
-
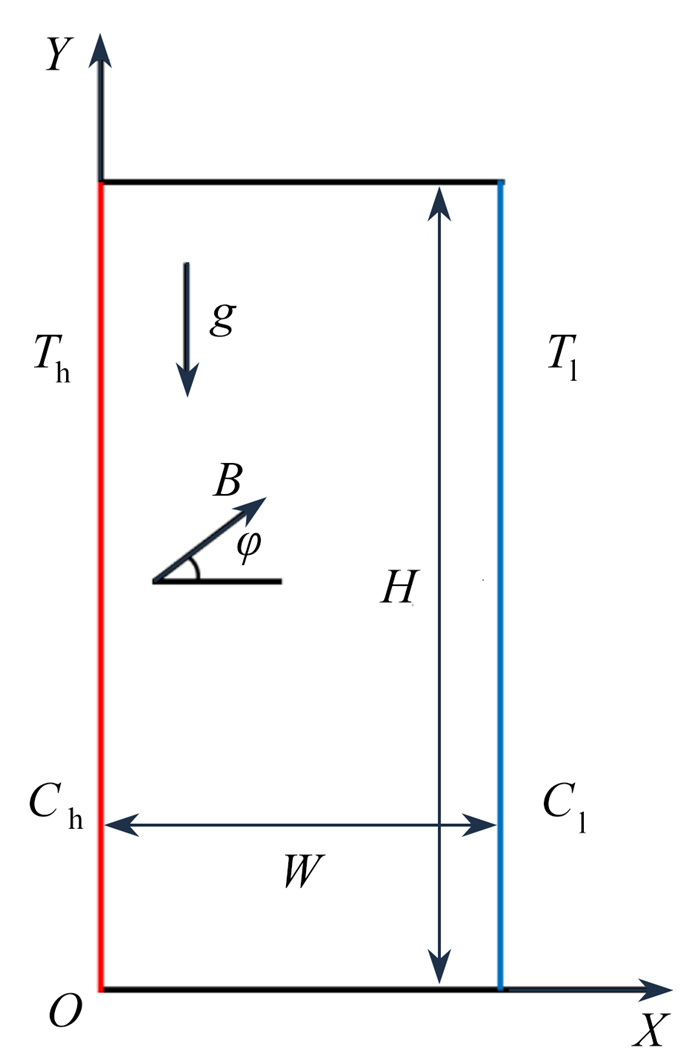
摘要: 采用高精度、高分辨率的数值方法，对三种方向的外磁场下长腔内不同液态金属双扩散对流的动力学特性进行直接数值模拟研究，揭示了流体物性参数Prandtl数(Pr)、磁场方向以及磁场强度对流动和传热传质的影响规律.结果表明：在所考察的Prandtl数范围内，随着Pr的增大，弱磁场时，流动从非定常的周期流动过渡为定常对流，其中Prandtl数为0.03时该对流系统存在非定常解，流动是周期性的，传热传质效率先快速增长，之后增速变缓；中等强度磁场时，流动始终是定常的，传热传质效率随Prandtl数增大的增速进一步减缓；强磁场时，流动总是定常的，传热传质效率几乎不随Prandtl数的改变而改变.在同一磁场强度下，相比于45°倾斜磁场和水平磁场，垂直磁场对传热传质效率产生的抑制作用较小.Abstract: With the high-precision and high-resolution numerical method, the dynamics of double-diffusive convection of different liquid metals in a long cavity under external magnetic fields in 2 directions was directly simulated, to reveal the influences of the fluid property parameter of Prandtl number Pr, the magnetic field direction and the magnetic field intensity on the flow and the heat and mass transfers. The results indicate that, within the range of the considered Pr values, the flow transitions from periodic to steady with the increase of Pr for weak magnetic fields. Specifically, when the Pr is 0.03, the convective system will have unsteady solution, and the flow will be periodic. The efficiency of heat and mass transfers initially increase rapidly, then slowly. For the moderately strong magnetic field, the flow remains steady, and the growth rate of the heat and mass transfer efficiency slows down further with Pr increasing. For the strong magnetic field, the flow is always steady, and the efficiency of the heat and mass transfer hardly changes with the Pr. Under the same magnetic field intensity, compared with the inclined magnetic field with a direction of 45° and the horizontal magnetic field, the vertical magnetic field has a weaker suppressive effect on the heat and mass transfer efficiency.
-
图 2 弱磁场(Ha=10)下传热传质随Prandtl数的变化
注 为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.
Figure 2. Variations of heat and mass transfers with the Prandtl number in a weak magnetic field (Ha=10)
图 3 Pr=0.03时，一个周期内流场结构的演变
Figure 3. The evolution of the flow field structure in one cycle for Pr=0.03
图 4 Pr=0.03时，流场中各特征量的变化情况
Figure 4. Variations of the characteristic quantities in the flow field when Pr=0.03
图 5 中等强度磁场(Ha=30)下传热传质随Prandtl数的变化
Figure 5. Variations of heat and mass transfers with the Prandtl number in a medium intensity magnetic field (Ha=30)
图 6 强磁场(Ha=100)下传热传质随Prandtl数的变化
Figure 6. Variations of heat and mass transfers with the Prandtl number under a strong magnetic field (Ha=100)
图 7 不同磁场方向下传热传质随Hartmann数的变化(Pr=0.025)
Figure 7. Variations of heat and mass transfers with the Hartmann number for different magnetic field directions (Pr=0.025)
表 1 水平磁场φ=0°下的网格检验结果
Table 1. The grid independence test of φ=0°
grid umax error/% vmax error/% Nuav error/% Shav error/% 61×121 27.160 8 0.19 41.764 1 0.20 2.287 9 0.26 3.774 5 0.28 71×141 27.188 6 0.09 41.345 1 1.20 2.290 8 0.13 3.779 3 0.15 81×161 27.202 7 0.04 41.831 4 0.03 2.292 4 0.06 3.782 2 0.07 91×181 27.209 3 0.01 41.593 8 0.60 2.293 3 0.02 3.783 8 0.03 101×201 27.212 4 - 41.845 7 - 2.293 8 - 3.784 9 - 
下载: 导出CSV
表 2 水平磁场φ=45°下的网格检验结果
Table 2. The grid independence test of φ=45°
grid umax error/% vmax error/% Nuav error/% Shav error/% 61×121 27.964 0 0.40 42.488 5 0.35 2.315 0 1.00 3.816 9 0.24 71×141 27.995 5 0.29 42.083 4 1.30 2.318 2 0.86 3.822 3 0.10 81×161 28.011 9 0.23 42.563 3 0.17 2.319 9 0.79 3.825 3 0.02 91×181 28.020 1 0.20 42.336 3 0.70 2.320 9 0.75 3.827 1 0.03 101×201 28.075 4 - 42.635 6 - 2.338 4 - 3.826 1 -
下载: 导出CSV
表 3 水平磁场φ=90°下的网格检验结果
Table 3. The grid independence test of φ=90°
grid umax error/% vmax error/% Nuav error/% Shav error/% 61×121 29.554 4 0.43 43.528 6 2.37 2.357 9 0.31 3.879 7 0.32 71×141 29.591 8 0.31 43.123 3 1.17 2.361 5 0.16 3.885 7 0.17 81×161 29.611 5 0.24 43.613 6 0.04 2.363 5 0.07 3.889 1 0.08 91×181 29.651 5 0.11 43.386 5 0.56 2.364 5 0.03 3.891 1 0.03 101×201 29.682 6 - 43.631 9 - 2.365 2 - 3.892 3 -
下载: 导出CSV
-
[1] IHLI T, BASU T K, GIANCARLI L M, et al. Review of blanket designs for advanced fusion reactors[J]. Fusion Engineering and Design, 2008, 83(7/9): 912-919. [2] 倪明玖. 磁约束核聚变反应堆研发相关的金属流体力学问题研究[J]. 中国科学: 物理学 力学 天文学, 2013, 43(12): 1570-1578.NI Mingjiu. Liquid metal hydrodynamics relevant to R&D of magnetocondined fusion reactor[J]. Scientia Sinica: Physica, Mechanica & Astronomica, 2013, 43(12): 1570-1578. (in Chinese) [3] SUN Z H I, GUO M, VLEUGELS J, et al. Strong static magnetic field processing of metallic materials: a review[J]. Current Opinion in Solid State and Materials Science, 2012, 16(5): 254-267. doi: 10.1016/j.cossms.2012.08.001 [4] RUDRAIAH N, BARRON R M, VENKATACHALAPPA M, et al. Effect of a magnetic field on free convection in a rectangular enclosure[J]. International Journal of Engineering Science, 1995, 33(8): 1075-1084. doi: 10.1016/0020-7225(94)00120-9 [5] BURR U, MVLLER U. Rayleigh-Bénard convection in liquid metal layers under the influence of a vertical magnetic field[J]. Physics of Fluids, 2001, 13(11): 3247-3257. doi: 10.1063/1.1404385 [6] AURNOU J M, OLSON P L. Experiments on Rayleigh-Bénard convection, magnetoconvection and rotating magnetoconvection in liquid gallium[J]. Journal of Fluid Mechanics, 2001, 430: 283-307. doi: 10.1017/S0022112000002950 [7] NANDUKUMAR Y, PAL P. Oscillatory instability and routes to chaos in Rayleigh-Bénard convection: effect of external magnetic field[J]. EPL (Europhysics Letters), 2015, 112(2): 24003. doi: 10.1209/0295-5075/112/24003 [8] TASAKA Y, IGAKI K, YANAGISAWA T, et al. Regular flow reversals in Rayleigh-Bénard convection in a horizontal magnetic field[J]. Physical Review E, 2016, 93: 043109. doi: 10.1103/PhysRevE.93.043109 [9] YU P X, XIAO Z, WU S, et al. High accuracy numerical investigation of double-diffusive convection in a rectangular cavity under a uniform horizontal magnetic field and heat source[J]. International Journal of Heat and Mass Transfer, 2017, 110: 613-628. doi: 10.1016/j.ijheatmasstransfer.2017.03.068 [10] NAFFOUTI A, BEN-BEYA B, LILI T. Three-dimensional Rayleigh-Bénard magnetoconvection: effect of the direction of the magnetic field on heat transfer and flow patterns[J]. Comptes Rendus Mécanique, 2014, 342(12): 714-725. doi: 10.1016/j.crme.2014.09.001 [11] SELIMLI S, RECEBLI Z, ARCAKLIOGLU E. Combined effects of magnetic and electrical field on the hydrodynamic and thermophysical parameters of magnetoviscous fluid flow[J]. International Journal of Heat and Mass Transfer, 2015, 86: 426-432. doi: 10.1016/j.ijheatmasstransfer.2015.02.074 [12] YU X X, ZHANG J, NI M J. Numerical simulation of the Rayleigh-Benard convection under the influence of magnetic fields[J]. International Journal of Heat and Mass Transfer, 2018, 120: 1118-1131. doi: 10.1016/j.ijheatmasstransfer.2017.11.151 [13] 虞培祥. 不可压导电流体流动问题的流函数-速度型算法研究及应用[D]. 上海: 复旦大学, 2013.YU Peixiang. Research and application of streamfunction-velocity algorithm for incompressible conductive fluid flow problems[D]. Shanghai: Fudan University, 2013. (in Chinese) [14] YANAGISAWA T, HAMANO Y, MIYAGOSHI T, et al. Convection patterns in a liquid metal under an imposed horizontal magnetic field[J]. Physical Review E, 2013, 88(6): 063020. doi: 10.1103/PhysRevE.88.063020 [15] BUSSE F H. Asymptotic theory of wall-attached convection in a horizontal fluid layer with a vertical magnetic field[J]. Physics of Fluids, 2008, 20(2): 024102. doi: 10.1063/1.2837175 [16] 仇建新. 外磁场作用下饱和多孔介质中液态金属流动与传热特性数值模拟[D]. 上海: 复旦大学, 2017.QIU Jianxin. Numerical simulation of liquid metal flow and heat transfer characteristics in saturated porous media under external magnetic field[D]. Shanghai: Fudan University, 2017. (in Chinese) [17] LIU W J, KRASNOV D, SCHUMACHER J. Wall modes in magnetoconvection at high Hartmann numbers[J]. Journal of Fluid Mechanics, 2018, 849: R2. doi: 10.1017/jfm.2018.479 [18] LIM Z L, CHONG K L, DING G Y, et al. Quasistatic magnetoconvection: heat transport enhancement and boundary layer crossing[J]. Journal of Fluid Mechanics, 2019, 870: 519-42. doi: 10.1017/jfm.2019.232 [19] YAN M, CALKINS M A, MAFFEI S, et al. Heat transfer and flow regimes in quasi-static magnetoconvection with a vertical magnetic field[J]. Journal of Fluid Mechanics, 2019, 877: 1186-1206. doi: 10.1017/jfm.2019.615 [20] ZVRNER T, SCHINDLER F, VOGT T, et al. Flow regimes of Rayleigh-Bénard convection in a vertical magnetic field[J]. Journal of Fluid Mechanics, 2020, 894: A21. doi: 10.1017/jfm.2020.264 [21] TASAKA Y, YANAGISAWA T, FUJITA K, et al. Two-dimensional oscillation of convection roll in a finite liquid metal layer under a horizontal magnetic field[J]. Journal of Fluid Mechanics, 2021, 911: A19. doi: 10.1017/jfm.2020.1047 [22] BENDARAA A, CHARAFI M M, HASNAOUI A. Numerical modeling of natural convection in horizontal and inclined square cavities filled with nanofluid in the presence of magnetic field[J]. The European Physical Journal Plus, 2019, 134(9): 468. doi: 10.1140/epjp/i2019-12814-8 [23] MOOLYA S, SATHEESH A. Role of magnetic field and cavity inclination on double diffusive mixed convection in rectangular enclosed domain[J]. International Communications in Heat and Mass Transfer, 2020, 118: 104814. doi: 10.1016/j.icheatmasstransfer.2020.104814 [24] REDDY N, MURUGESAN K. Magnetic field influence on double-diffusive natural convection in a square cavity: a numerical study[J]. Numerical Heat Transfer (Part A): Applications, 2017, 71(4): 448-475. doi: 10.1080/10407782.2016.1277922 [25] YANG J Q, ZHAO B X. Numerical investigation of double-diffusive convection in rectangular cavities with different aspect ratio Ⅰ: high-accuracy numerical method[J]. Computers & Mathematics With Applications, 2021, 94: 155-169. [26] ZHAO B X, YANG J Q. Numerical investigation of 2D double-diffusive convection in rectangular cavities with different aspect ratios: heat and mass transfer and flow characteristics[J]. Physics of Fluids, 2022, 34(3): 034120. doi: 10.1063/5.0084537 -
计量
- 文章访问数: 43
- HTML全文浏览量: 19
- PDF下载量: 15
- 被引次数: 0
渝公网安备50010802005915号