Analysis on Stress Singularity of Plane Joints With the Differential Quadrature Method
-
摘要:
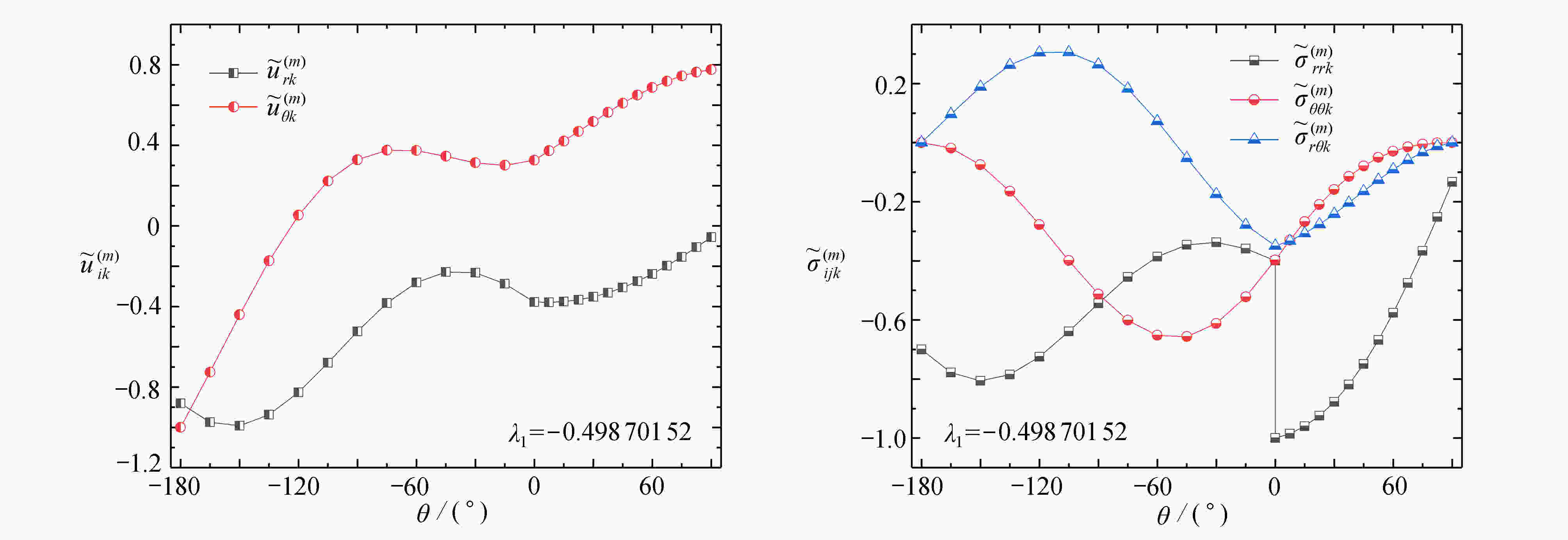
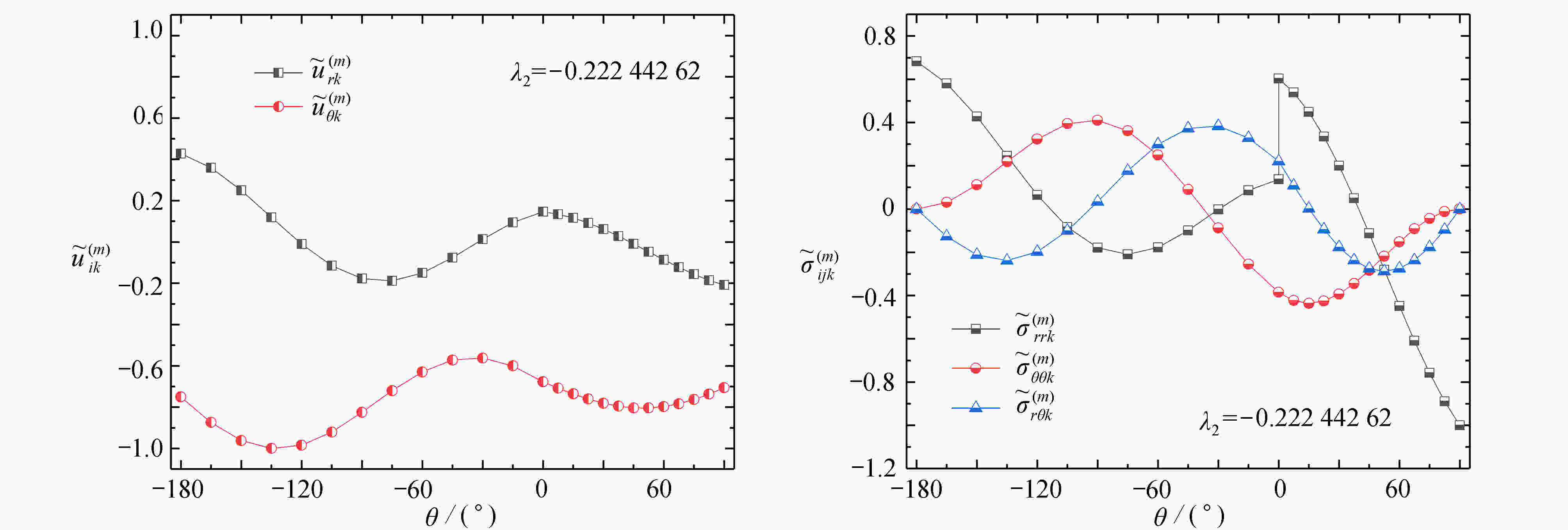
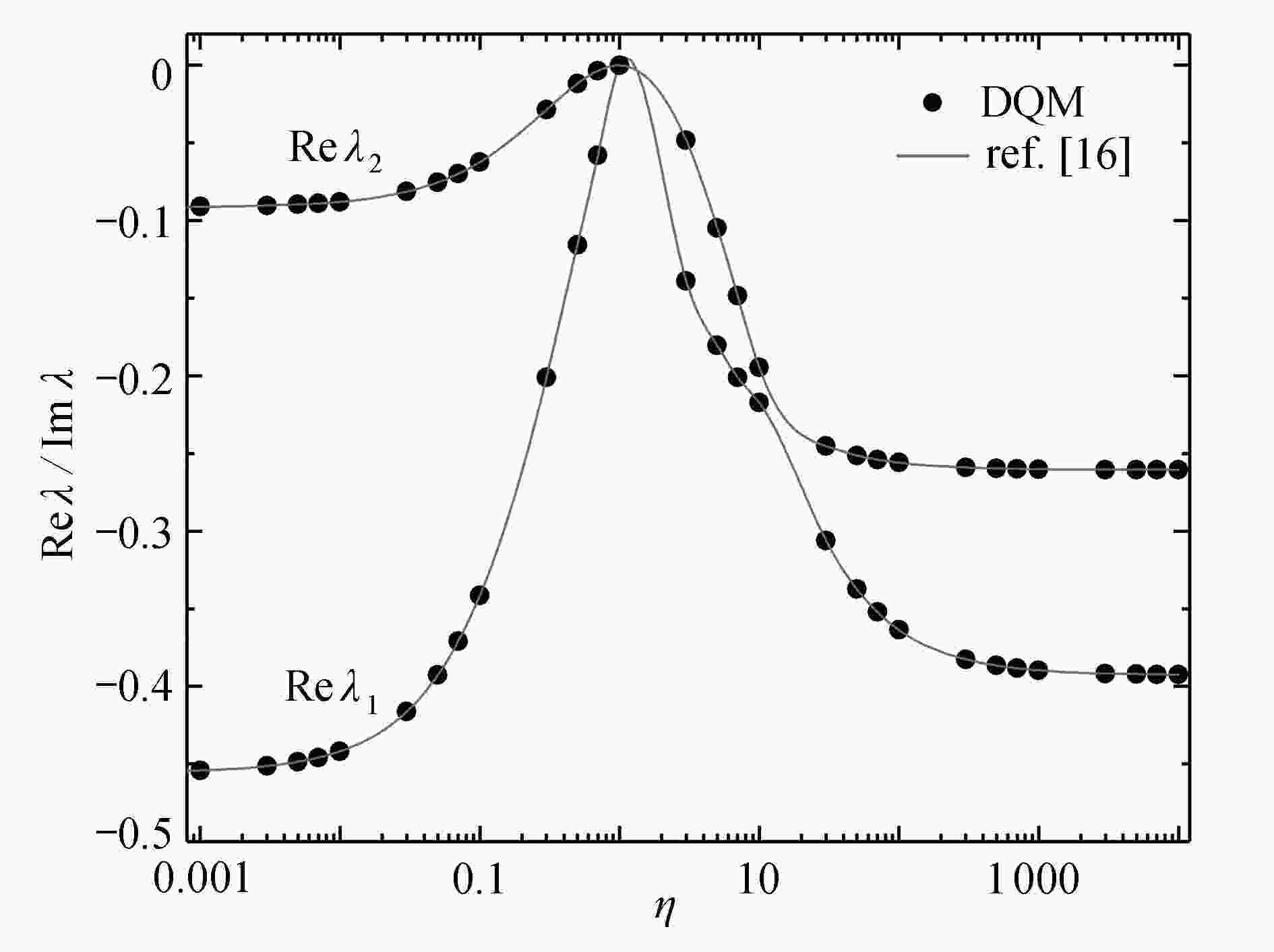
对于双材料平面接头问题提出了一个分析应力奇性指数的新方法:微分求积法(DQM)。首先,将平面接头连接点处位移场的径向渐近展开格式代入平面弹性力学控制方程,获得了关于应力奇性指数的常微分方程组(ODEs)特征值问题。然后,基于DQM理论,将ODEs的特征值问题转化为标准型广义代数方程组特征值问题,求解之可一次性地计算出双材料平面接头连接点处应力奇性指数,同时,一并求出了接头连接点处相应的位移和应力特征函数。数值计算结果说明该文DQM计算平面接头连接点处应力奇性指数的结果是正确的。
Abstract:A novel differential quadrature method (DQM) for analysis of the stress singularity index was proposed. Firstly, the radial asymptotic expansion scheme of the displacement field at the connection point of the plane joint was substituted into the governing equation of plane elasticity, and the eigenvalue problem of ordinary differential equations (ODEs) about the stress singularity index was obtained. Then, based on the DQM theory, the eigenvalue problem of ordinary differential equations was transformed into the eigenvalue problem of standard generalized algebraic equations. The stress singularity index at the connection point of the bi-material plane joint was calculated at one time, and the corresponding displacement and stress characteristic functions at the connection point were obtained at the same time. The numerical results show that, the DQM is correct in calculation of the stress singularity index at the connection point of the plane joint.
-
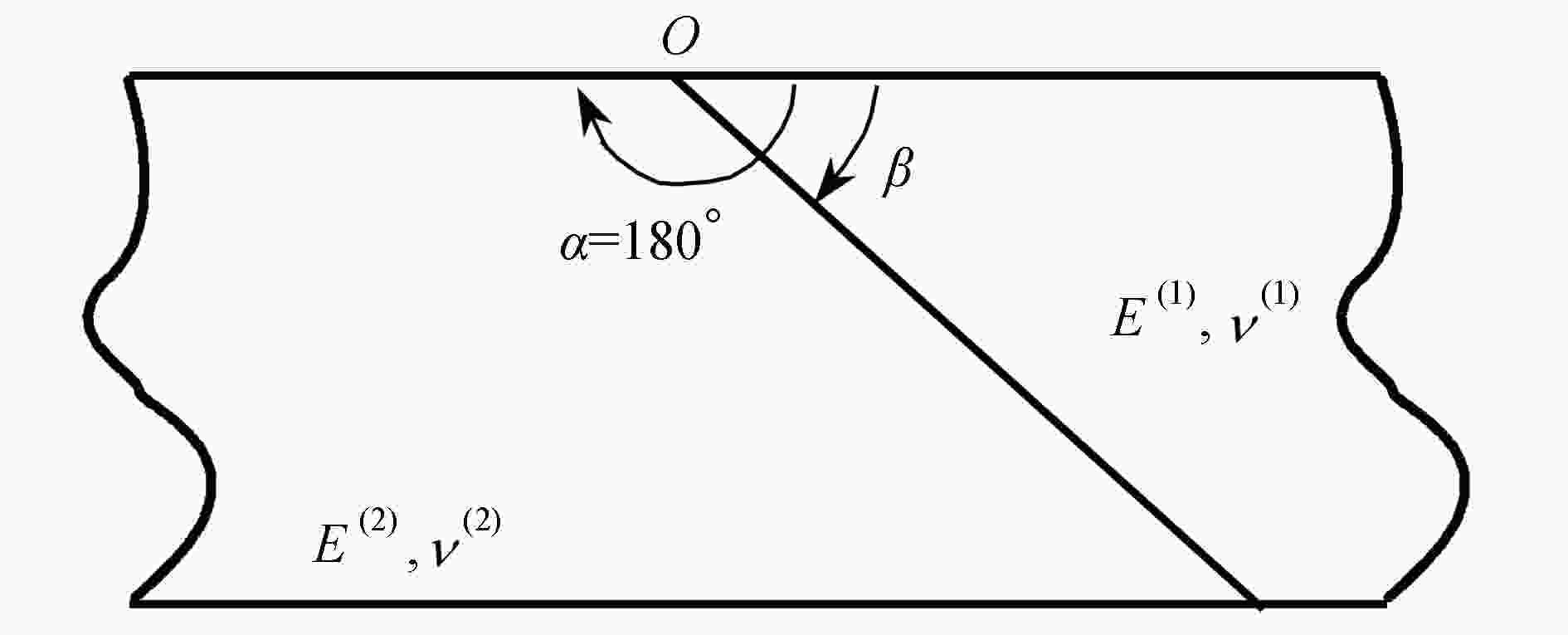
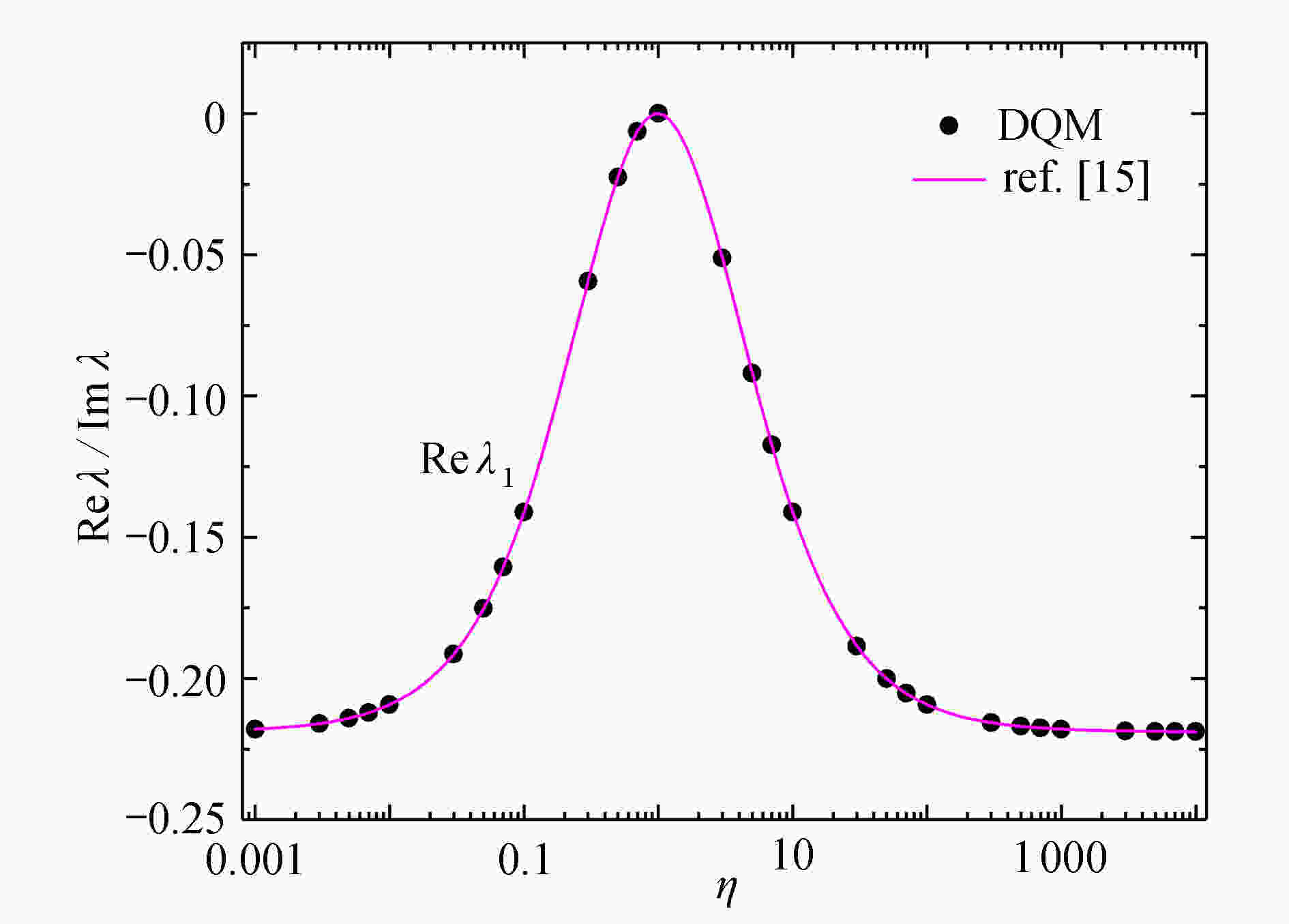
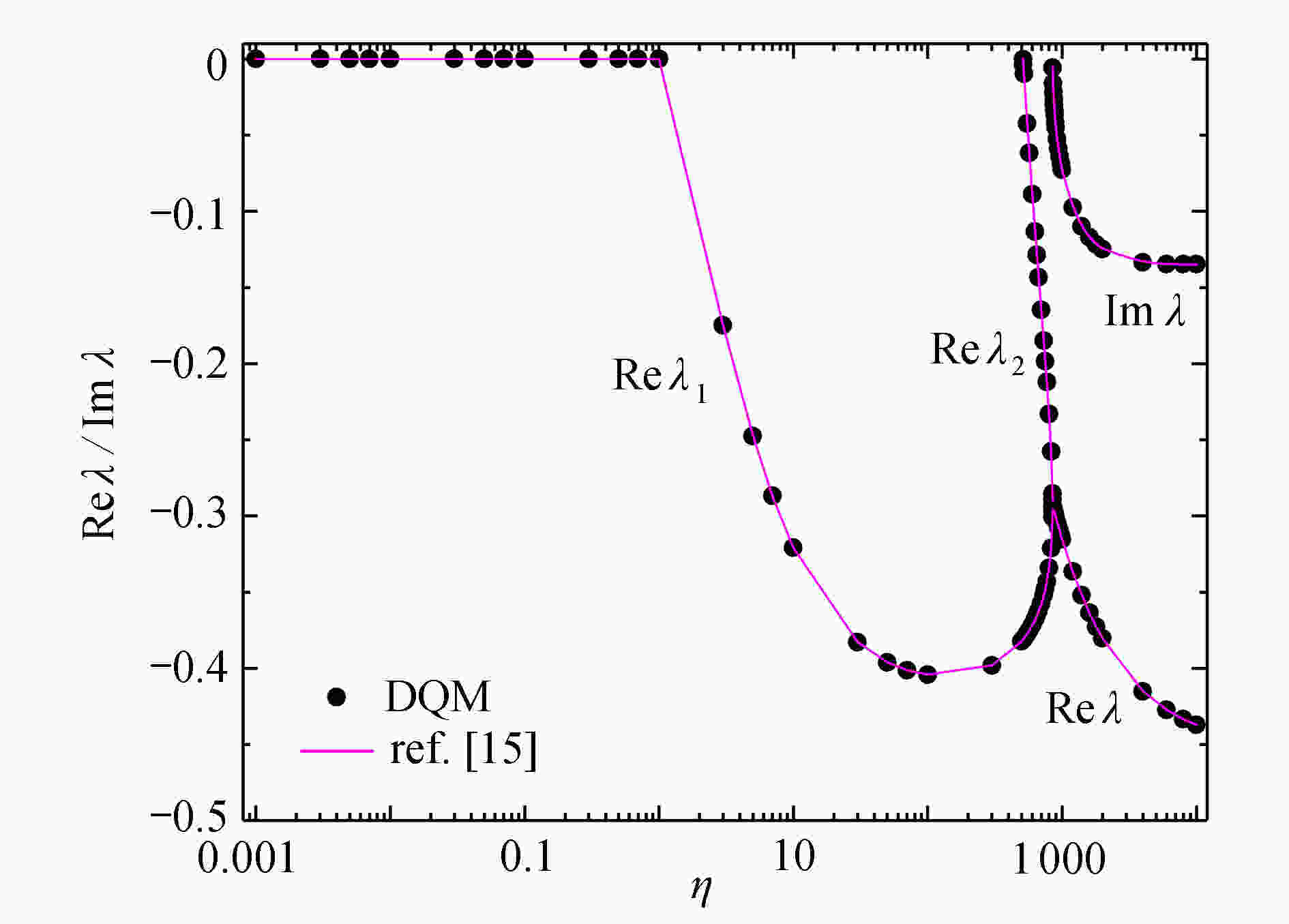
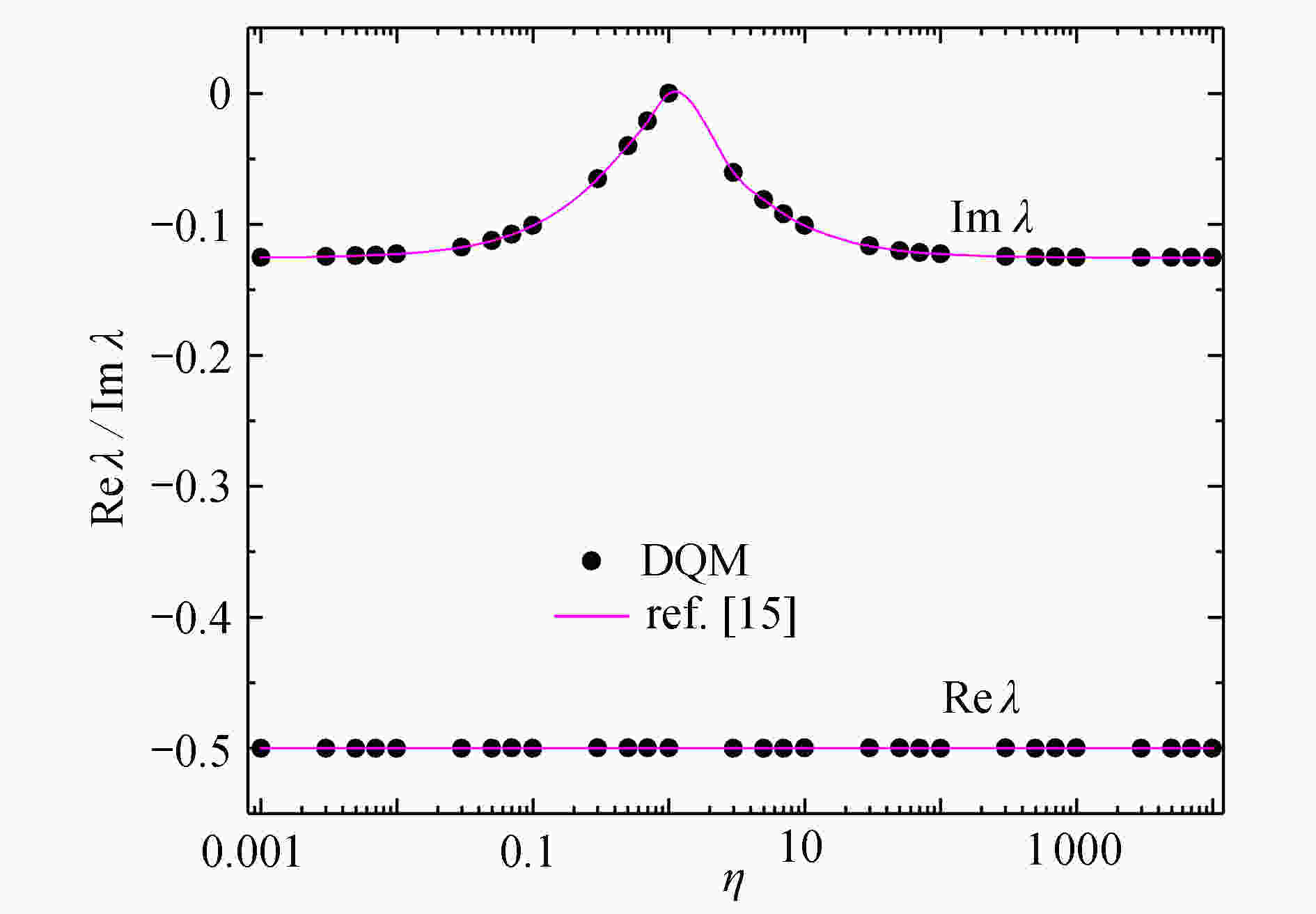
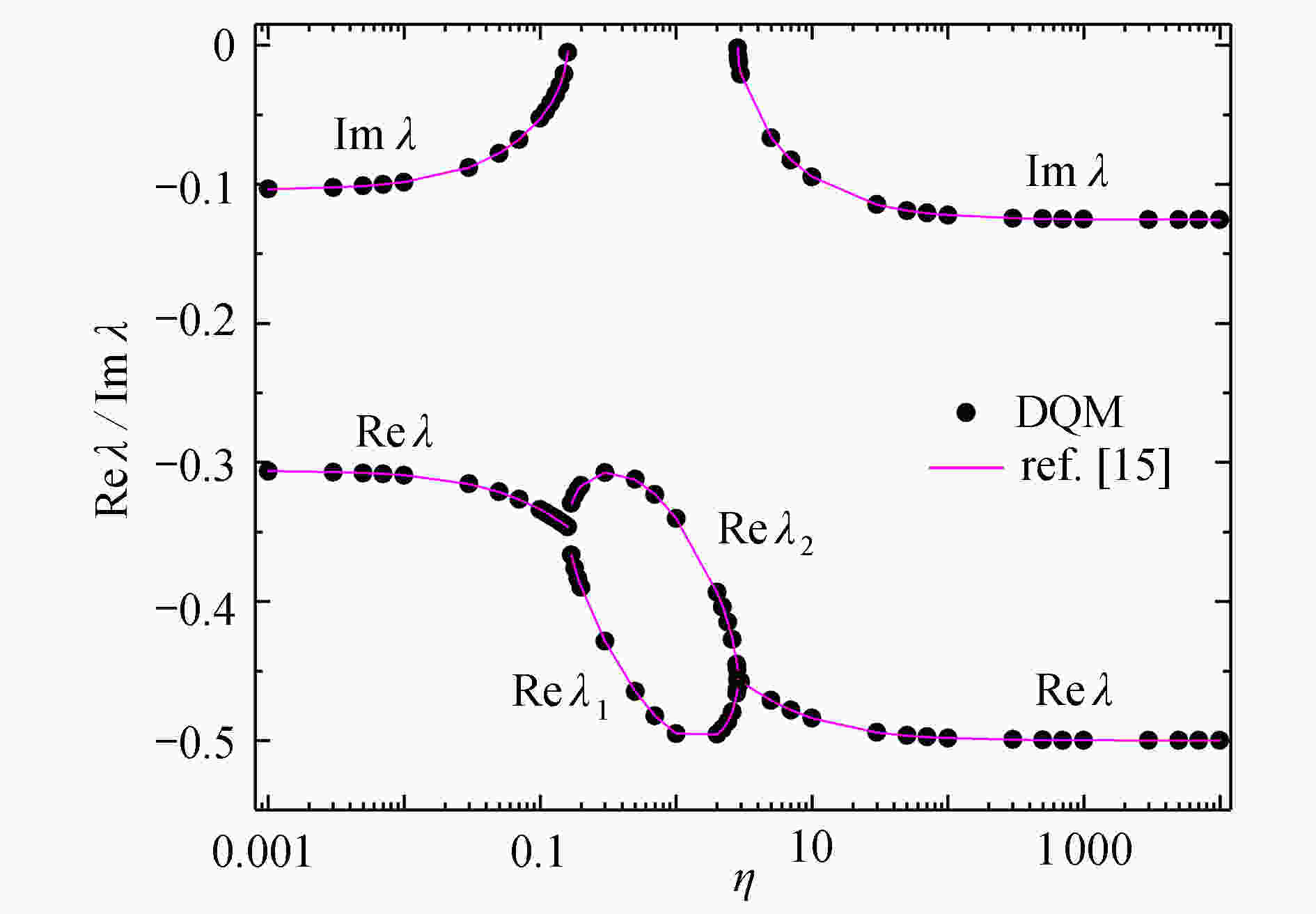
表 1 η = 3.0时,平面接头模型1的第1阶应力奇性指数λ1计算值随离散单元数N的变化
Table 1. Variation of the 1st-order stress singularity index of plane joint model 1 with number of discrete elements N for η = 3.0
β/(°) η = 3.0 N = 4 N = 6 N = 8 N = 10 N = 12 ref. [14] 15 −0.142 935 841 −0.171 926 062 −0.172 359 547 −0.174 841 720 −0.174 843 563 −0.174 837 30 −0.172 189 244 −0.168 084 637 −0.168 357 284 −0.171 008 990 −0.171 010 442 −0.171 006 45 −0.120 351 300 −0.097 236 818 −0.097 575 977 −0.100 173 229 −0.100 174 236 −0.100 170 60 −0.042 900 476 −0.008 193 459 −0.008 995 020 −0.011 148 934 −0.011 150 916 −0.011 146 75 −0.039 048 671 −0.009 146 856 −0.011 225 252 −0.012 133 752 −0.012 128 895 −0.012 132 90 −0.072 175 519 −0.480 812 299 −0.050 550 470 −0.051 075 515 −0.051 073 963 −0.051 074 表 2 η = 4.0时,平面接头模型1的第1阶应力奇性指数λ1计算值随离散单元数N的变化
Table 2. Variation of the 1st-order stress singularity index of plane joint model 1 with number of discrete elements N for η = 4.0
β/(°) η = 4.0 N = 4 N = 6 N = 8 N = 10 N = 12 ref. [14] 15 −0.191 925 955 −0.214 512 897 −0.215 023 699 −0.217 433 336 −0.217 435 029 −0.217 430 30 −0.205 239 132 −0.198 498 506 −0.198 833 494 −0.201 426 794 −0.201 428 700 −0.201 424 45 −0.136 422 364 −0.112 082 716 −0.112 388 798 −0.115 019 309 −0.115 020 289 −0.115 016 60 −0.042 586 733 −0.006 382 054 −0.007 046 986 −0.009 334 714 −0.009 339 771 −0.009 332 75 −0.056 361 125 −0.029 365 948 −0.031 589 068 −0.032 355 253 −0.032 357 518 −0.032 354 90 −0.092 606 092 −0.071 003 905 −0.073 550 960 −0.073 999 146 −0.073 998 571 −0.073 998 表 3 η = 5.0时,平面接头模型1的第1阶应力奇性指数λ1计算值随离散单元数N的变化
Table 3. Variation of the 1st-order stress singularity index of plane joint model 1 with number of discrete elements N for η = 5.0
β/(°) η = 5.0 N = 4 N = 6 N = 8 N = 10 N = 12 ref. [14] 15 −0.226 230 590 −0.244 514 386 −0.245 073 248 −0.247 438 211 −0.247 439 135 −0.247 435 30 −0.226 503 691 −0.218 078 739 −0.218 450 970 −0.221 009 453 −0.221 009 756 −0.221 006 45 −0.145 347 760 −0.120 250 154 −0.120 521 489 −0.123 186 475 −0.123 187 448 −0.123 183 60 −0.039 263 008 −0.001 610 141 −0.002 117 127 −0.004 559 442 −0.004 551 847 −0.004 556 75 −0.073 518 508 −0.049 300 481 −0.051 647 322 −0.052 291 762 −0.052 286 395 −0.052 290 90 −0.108 633 756 −0.088 879 155 −0.091 481 239 −0.091 875 053 −0.091 874 796 −0.091 874 -
[1] LIU C I, CHUE C H. On the stress singularity of dissimilar anisotropic wedges and junctions in anti-plane shear[J]. Composite Structures, 2006, 73(4): 432-442. doi: 10.1016/j.compstruct.2005.02.015 [2] 张志春, 强洪夫, 周伟. 基于粘结界面模型的三维裂纹扩展研究[J]. 计算物理, 2010, 27(4): 586-592. (ZHANG Zhichun, QIANG Hongfu, ZHOU Wei. 3D crack propagation in cohesive zone models[J]. Chinese Journal of Computational Physics, 2010, 27(4): 586-592.(in Chinese) doi: 10.3969/j.issn.1001-246X.2010.04.015 [3] HEIN V L, ERDOGAN F. Stress singularities in a two-material wedge[J]. International Journal of Fracture, 1971, 7: 317-330. doi: 10.1007/BF00184307 [4] BOGY D B. On the problem of edgebonded elastic quarteroplanes loaded at the boundary[J]. International Journal of Solids & Structures, 1970, 6(9): 1287-1313. [5] BOGY D B. Two edge-bonded elastic wedges of different materials and wedge angles under surface tractions[J]. Journal of Applied Mechanics, 1971, 38(2): 377-386. doi: 10.1115/1.3408786 [6] WILLIAMS M L. Stress singularities resulting from various boundary conditions in angular corners of plates in extension[J]. Journal of Applied Mechanics, 1952, 19(4): 526-528. doi: 10.1115/1.4010553 [7] ENGLAND A H. On stress singularities in linear elasticity[J]. International Journal of Engineering Science, 1971, 9(6): 571-585. doi: 10.1016/0020-7225(71)90039-5 [8] STERN M, SONI M L. On the computation of stress intensities at fixed-free corners[J]. International Journal of Solids & Structures, 1976, 12(5): 331-337. [9] CARPENTER W C, BYERS C. A path independent integral for computing stress intensities for V-notched cracks in a bi-material[J]. International Journal of Fracture, 1987, 35: 245-268. doi: 10.1007/BF00276356 [10] PAGGI M, CARPINTERI A. On the stress singularities at multi-material interfaces and related analogies with fluid dynamics and diffusion[J]. Applied Mechanics Reviews, 2008, 61(2): 1-22. [11] PICU C R, GUPTA V. Stress singularities at triple junctions with freely sliding grains[J]. International Journal of Solids & Structures, 1996, 33(11): 1535-1541. [12] LIU X H, SUO Z, MA Q. Split singularities: stress field near the edge of a silicon die on a polymer substrate[J]. Computer Methods in Applied Mechanics and Engineering, 1999, 47(1): 67-76. [13] BONIFACE V, SIMHA K R Y. Suppression of complex singularity using wedge interphase in interface fracture[J]. International Journal of Solids and Structure, 2001, 38(30/31): 5411-5420. [14] 张金轮, 葛仁余, 韩有民, 等. 各向同性材料接头和界面相交裂纹应力奇异性特征分析[J]. 应用力学学报, 2017, 34(1): 14-19. (ZHANG Jinlun, GE Renyu, HAN Youmin, et al. Analysis of the stress singularity of the junctions and plane cracks terminating at the interface in bonded dissimilar isotropic materials[J]. Chinese Journal of Applied Mechanics, 2017, 34(1): 14-19.(in Chinese) [15] SATOR C, BECKER W. Closed-form solutions for stress singularities at plane bi- and trimaterial junctions[J]. Archive of Applied Mechanics, 2012, 82(5): 643-658. doi: 10.1007/s00419-011-0580-6 [16] CARPINTERI A, PAGGI M. Analytical study of the singularities arising at multi-material interfaces in 2D linear elastic problems[J]. Engineering Fracture Mechanics, 2007, 74(1/2): 59-74. [17] CHO S B, CARPENTER W C. The complex potential approach to power-logarithmic stress singularities for V-notched cracks in a bi-material[J]. KSME International Journal, 1999, 13(1): 19-25. doi: 10.1007/BF02946119 [18] BELLMAN R, CASTI J. Differential quadrature and long-term integration[J]. Journal of Mathematical Analysis and Applications, 1971, 34: 235-238. doi: 10.1016/0022-247X(71)90110-7 [19] BERT C W, WANG X, STRIZ A G. Differential quadrature for static and free vibration analysis of anisotropic plates[J]. International Journal of Solids and Structures, 1993, 30(13): 1737-1744. doi: 10.1016/0020-7683(93)90230-5 [20] BERT C W, JANG S K, STRIZ A G. Two new approximate methods for analyzing free vibration of structural components[J]. AIAA Journal, 2015, 26(5): 612-618. [21] LIEW K M, TEO T M, HAN J B. Three-dimensional static solutions of rectangular plates by variant differential quadrature method[J]. International Journal of Mechanical Sciences, 2001, 43(7): 1611-1628. doi: 10.1016/S0020-7403(00)00098-9 [22] YANG J, KITIPORNCHAI S, LIEW K M. Non-linear analysis of thermos-electro-mechanical behavior of shear deformable FGM plates with piezoelectric actuators[J]. International Journal for Numerical Methods in Engineering, 2004, 59(12): 1605-1632. doi: 10.1002/nme.932 [23] 许金泉. 界面力学[M]. 1版. 北京: 科学出版社, 2006.XU Jinquan. The Mechanics of Interface[M]. 1st ed. Beijing: Science Press, 2006. (in Chinese) -





 下载:
下载:






 渝公网安备50010802005915号
渝公网安备50010802005915号