A High-Order Finite Difference Scheme for 3D Unsteady Convection Diffusion Reaction Equations
-
摘要:
针对三维非稳态对流扩散反应方程,构造了一种高精度紧致有限差分格式,对空间的离散采用四阶紧致差分方法,对时间的离散采用Taylor级数展开和余项修正技术,所提格式在时间上的精度为二阶、在空间上的精度为四阶。利用Fourier稳定性分析法证明了该格式是无条件稳定的。最后给出数值算例验证了理论结果。
-
关键词:
- 对流扩散反应方程 /
- 变对流项和反应项系数 /
- 高精度紧致格式 /
- 无条件稳定 /
- 有限差分法
Abstract:Based on the 4th-order compact difference scheme for spatial discretization, the Taylor series expansion and the error remainder correction method for temporal discretization, a high-order compact finite difference scheme for solving the 3D unsteady convection diffusion reaction equations was proposed. The unconditional stability was proved with the Fourier analysis method. The proposed scheme has 2nd-order accuracy in time and 4th-order accuracy in space. At last, numerical examples validate the theoretical results.
-
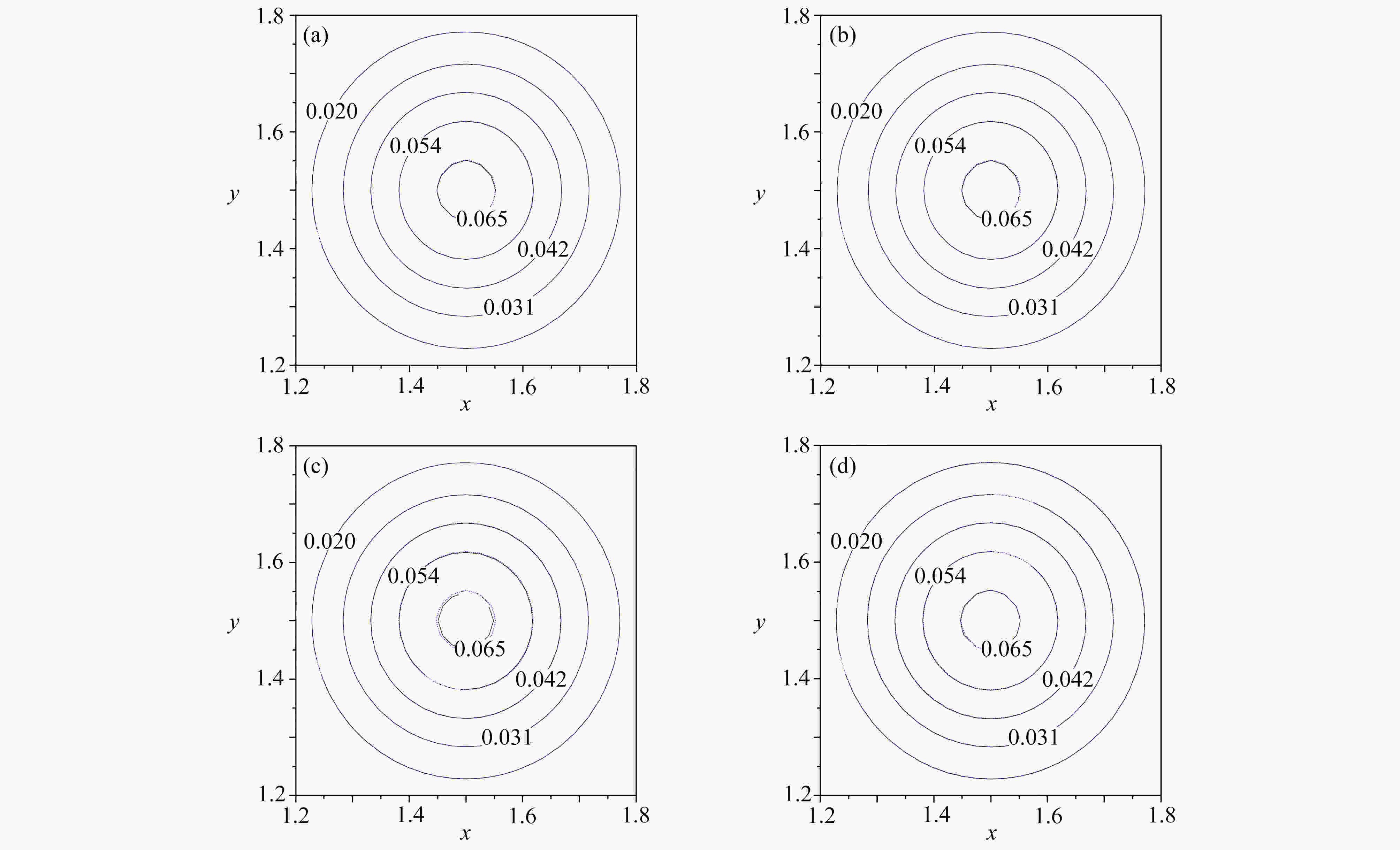
图 1 问题2当
$ h = 0.025{\text{,}}t = 1.25{\text{,}}p = q = r = 0.8{\text{,}}\tau = 6.25 \times {10^{ - 3}} $ 时,在区域$ 1.2 \leqslant x,y \leqslant 1.8 $ 内,截面$ z = 1.5 $ 上的等值线图:(a) PHOC-ADI格式和精确解;(b) EHOC-ADI格式和精确解;(c) RHOC-ADI格式和精确解;(d) 本文格式和精确解Figure 1. Numerical contour lines compared with exact solutions at
$ z = 1.5 $ in region$ 1.2 \leqslant x,y \leqslant 1.8 $ in problem 2 for$ h = 0.025,\;t = 1.25,$ $p = q = r = 0.8{\text{,}}\;\tau = 6.25 \times {10^{ - 3}}$ : (a) PHOC-ADI scheme and exact solutions; (b) EHOC-ADI scheme and exact solutions; (c) RHOC-ADI scheme and exact solutions; (d) the present scheme and exact solutions图 2 问题2当
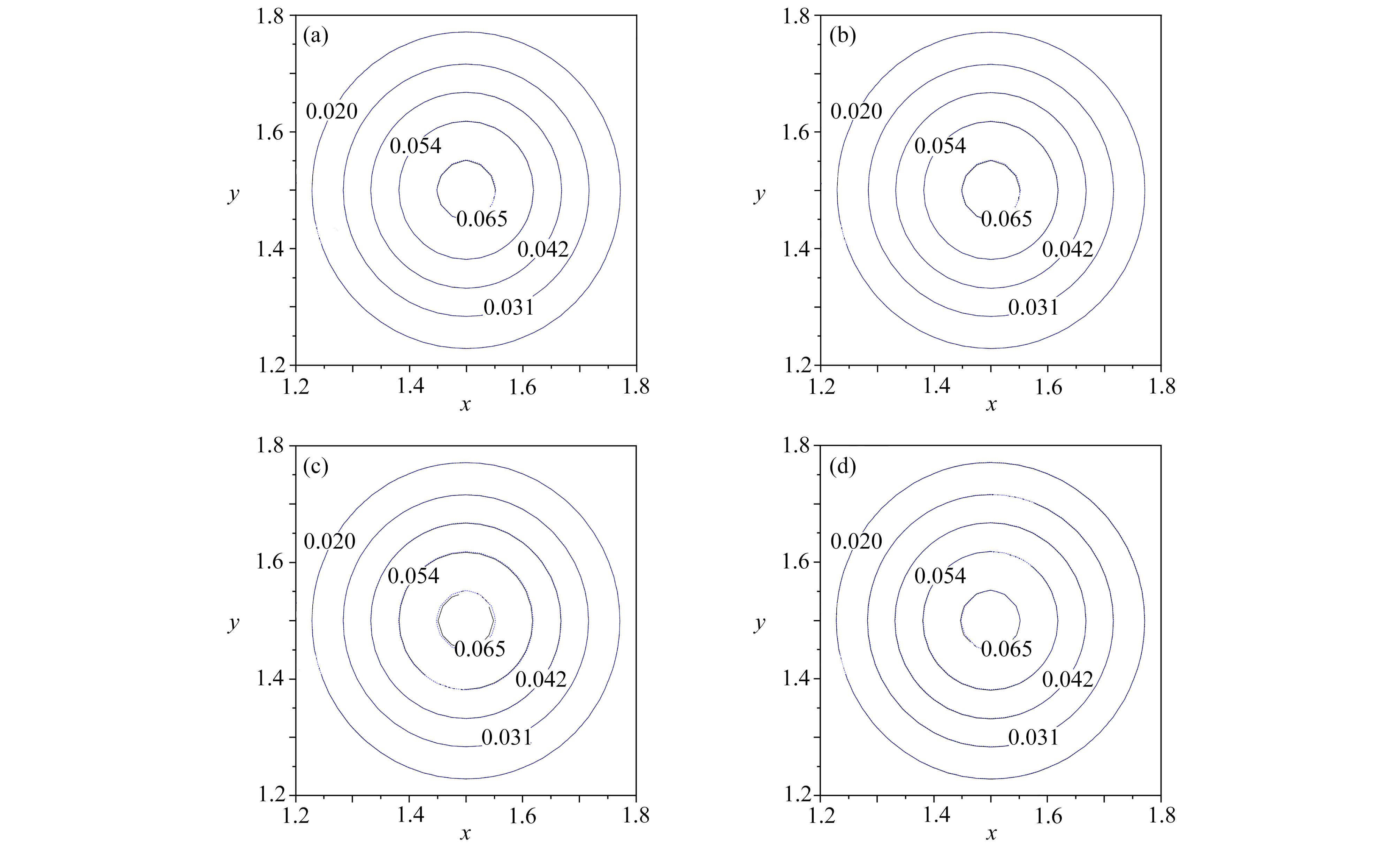
$h = 0.025,\;t = 1.25 \times {10^{-4}},\;p = q = r = 8\;000,\;\tau = 6.25 \times {10^{ - 7}}$ 时,在区域$ 1.2 \leqslant x,y \leqslant 1.8 $ 内,截面z=1.5上的等值线图:(a) PHOC-ADI格式和精确解;(b) EHOC-ADI格式和精确解;(c) RHOC-ADI格式和精确解;(d) 本文格式和精确解Figure 2. Numerical contour lines compared with exact solutions at
${{z}} = 1.5$ in region$ 1.2 \leqslant x,y \leqslant 1.8 $ in problem 2 for$h = 0.025,\;t = 1.25 \times {10^{-4}},\; $ $ p = q = r = 8\;000,\;\tau = 6.25 \times {10^{ - 7}}$ : (a) PHOC-ADI scheme and exact solutions; (b) EHOC-ADI scheme and exact solutions; (c) RHOC-ADI scheme and exact solutions;(d) the present scheme and exact solutions表 1 问题1当
$\tau = {h^2}, t = 0.25$ 时的$ {L_\infty } $ 误差和$ {L_2} $ 误差及收敛阶Table 1.
$ {L_\infty } $ errors,$ {L_2} $ errors and convergence rates for$\tau = {h^2},\;t = 0.25$ in problem 1$ p,q,r $ $ h $ C-N scheme BTCS scheme present scheme $ {L_\infty } $ $\delta $ $ {L_2} $ $\delta $ $ {L_\infty } $ $\delta $ $ {L_2} $ $\delta $ $ {L_\infty } $ $\delta $ $ {L_2} $ $\delta $ case ① 1/8 1.60E−2 5.62E−3 1.63E−2 5.73E−3 2.74E−4 1.67E−4 1/16 3.97E−3 2.01 1.40E−3 2.01 4.05E−3 2.00 1.42E−3 2.00 7.81E−6 5.13 3.87E−6 5.43 1/32 9.90E−4 2.00 3.48E−4 2.00 1.01E−3 2.00 3.56E−4 2.00 3.94E−7 4.30 1.62E−7 4.57 1/64 2.47E−4 2.00 8.71E−5 2.00 2.53E−4 2.00 8.89E−5 2.00 2.29E−8 4.10 8.08E−9 4.32 case ② 1/8 1.58E−2 5.55E−3 1.62E−2 5.66E−3 2.83E−4 1.70E−4 1/16 3.94E−3 2.00 1.38E−3 2.01 4.02E−3 2.01 1.41E−3 2.01 8.48E−6 5.06 4.10E−6 5.37 1/32 9.83E−4 2.00 3.44E−4 2.00 1.00E−3 2.01 3.52E−4 2.00 4.30E−7 4.30 1.76E−7 4.54 1/64 2.46E−4 2.00 8.61E−5 2.00 2.51E−4 1.99 8.79E−5 2.00 2.52E−8 4.09 9.75E−9 4.17 表 2 问题1当
$h = 0.031\;25, t = 0.5$ 时,本文格式的$ {L_\infty } $ 误差、$ {L_2} $ 误差、收敛阶及CPU时间Table 2.
$ {L_\infty } $ errors,$ {L_2} $ errors, convergence rates and CPU time for$h = 0.031\;25,\;t = 0.5$ in problem 1$ p,q,r $ $ \tau $ $ {L_\infty } $ $\delta $ $ {L_2} $ $\delta $ CPU time tCPU/s case ① 0.1 4.883E−4 1.654E−4 195.934 0.05 1.234E−4 1.98 4.076E−5 2.01 275.417 0.025 3.155E−5 1.97 1.050E−5 1.96 334.355 0.0125 8.050E−6 1.97 2.695E−6 1.96 406.406 0.00625 2.296E−6 1.81 7.981E−7 1.76 455.322 case ② 0.1 8.220E−4 2.528E−4 240.216 0.05 2.017E−4 2.03 6.189E−5 2.03 345.248 0.025 5.056E−5 2.00 1.550E−5 2.00 426.347 0.0125 1.292E−5 1.97 3.920E−6 1.98 520.264 0.00625 3.519E−6 1.88 1.039E−6 1.92 539.716 表 3 问题1当
$h = {1 \mathord{\left/ {\vphantom {1 {32}}} \right. } {32}}, t = 1$ 时,对不同网格比$\lambda = {\tau \mathord{/ {\vphantom {\tau {{h^2}}}} } {{h^2}}}$ 的$ {L_\infty } $ 误差和$ {L_2} $ 误差Table 3.
$ {L_\infty } $ errors and$ {L_2} $ errors for$h = {1 \mathord{\left/ {\vphantom {1 {32}}} \right. } {32}}{\text{,}}\;t = 1$ for different mesh ratio$\lambda = {\tau \mathord{/ {\vphantom {\tau {{h^2}}}} } {{h^2}}}$ values in problem 1$ p,q,r $ $ \lambda $ number of steps in the time direction C-N scheme BTCS scheme present scheme $ {L_\infty } $ $ {L_2} $ $ {L_\infty } $ $ {L_2} $ $ {L_\infty } $ $ {L_2} $ case ① 0.8 1280 2.044E−3 7.086E−4 2.079E−3 7.213E−4 8.703E−7 3.611E−7 1.6 640 2.043E−3 7.086E−4 2.114E−3 7.340E−4 9.715E−7 4.008E−7 3.2 320 2.043E−3 7.085E−4 2.185E−3 7.595E−4 1.495E−6 5.737E−7 6.4 160 2.043E−3 7.083E−4 2.327E−3 8.104E−4 3.841E−6 1.330E−6 case ② 0.8 1280 2.110E−3 7.362E−4 2.144E−3 7.485E−4 7.827E−7 3.002E−7 1.6 640 2.110E−3 7.362E−4 2.178E−3 7.608E−4 7.906E−7 2.496E−7 3.2 320 2.110E−3 7.364E−4 2.246E−3 7.854E−4 1.381E−6 4.394E−7 6.4 160 2.112E−3 7.371E−4 2.383E−3 8.345E−4 6.839E−6 2.142E−6 表 4 问题2当
$ h = 0.025 $ 时,在不同参数下的$ {L_\infty } $ 误差和$ {L_2} $ 误差Table 4.
$ {L_\infty } $ errors and$ {L_2} $ errors for$ h = 0.025 $ in problem 2scheme L∞ L2 $ t = 1.25,p = q = r = 0.8,\tau = 6.25 \times {10^{ - 3}} $ Douglas-Gunn ADI[1] 4.107E−3 5.674E−4 HOC-ADI[3] 1.454E−4 1.626E−5 PHOC-ADI[4] 1.444E−4 1.546E−5 EHOC-ADI[5] 1.044E−3 5.344E−5 RHOC-ADI[6] 1.039E−3 6.245E−5 present scheme 2.196E−4 3.434E−5 $ t = 1.25 \times {10^{ - 1}},p = q = r = 8,\tau = 6.25 \times {10^{ - 4}} $ Douglas-Gunn ADI[1] 1.963E−1 1.158E−2 HOC-ADI[3] 8.886E−2 3.530E−3 PHOC-ADI[4] 8.885E−2 3.529E−3 EHOC-ADI[5] 4.459E−2 1.531E−3 RHOC-ADI[6] 7.983E−3 3.777E−4 present scheme 1.566E−2 8.319E−4 $ t = 1.25 \times {10^{ - 2}},p = q = r = 80,\tau = 6.25 \times {10^{ - 5}} $ Douglas-Gunn ADI[1] 4.455E−1 2.285E−2 HOC-ADI[3] 3.010E−1 1.223E−2 PHOC-ADI[4] 3.018E−1 1.223E−2 EHOC-ADI[5] 1.375E−1 3.782E−3 RHOC-ADI[6] 2.674E−2 9.888E−4 present scheme 4.833E−2 1.939E−3 $ t = 1.25 \times {10^{ - 3}},p = q = r = 800,\tau = 6.25 \times {10^{ - 6}} $ Douglas-Gunn ADI[1] 4.874E−1 2.470E−2 HOC-ADI[3] 1.178E+20 4.617E+19 PHOC-ADI[4] 3.248E−1 1.411E−2 EHOC-ADI[5] 1.567E−1 4.199E−3 RHOC-ADI[6] 3.137E−2 1.115E−3 present scheme 5.562E−2 2.153E−3 $t = 1.25 \times {10^{ -4} },p = q = r = 8\;000,\tau = 6.25 \times {10^{ - 7} }$ Douglas-Gunn ADI[1] 4.918E−1 2.490E−2 HOC-ADI[3] 2.639E+24 1.523E+24 PHOC-ADI[4] 3.267E−1 1.433E−2 EHOC-ADI[5] 1.588E−1 4.244E−3 RHOC-ADI[6] 3.189E−2 1.129E−3 present scheme 5.642E−2 2.176E−3 表 5 问题2当
$ t = 0.25,\tau = {h^2},p = q = r = 0.8 $ 时,本文格式的$ {L_\infty } $ 误差和$ {L_2} $ 误差及收敛阶Table 5.
$ {L_\infty } $ errors,$ {L_2} $ errors and convergence rates for$ t = 0.25,\tau = {h^2},p = q = r = 0.8 $ in problem 2$ h $ $ {L_\infty } $ $\delta $ $ {L_2} $ $\delta $ 1/40 2.287E−4 2.861E−5 1/60 4.586E−5 3.96 5.516E−6 4.06 1/80 1.470E−5 3.95 1.730E−6 4.03 1/100 6.013E−6 4.01 7.057E−7 4.02 1/120 2.886E−6 4.03 3.396E−7 4.01 表 6 问题3当
$\tau = {h^2},\;\alpha = 0.1,\;t = 0.5$ 时的$ {L_\infty } $ 误差和$ {L_2} $ 误差及收敛阶Table 6.
$ {L_\infty } $ errors,$ {L_2} $ errors and convergence rates for$\tau = {h^2},\;\alpha = 0.1,\,t = 0.5$ in problem 3$ h $ DHOC[20] present scheme $ {L_\infty } $ $\delta $ $ {L_2} $ $\delta $ $ {L_\infty } $ $\delta $ $ {L_2} $ $\delta $ 1/8 9.079E−5 3.060E−5 5.848E−5 2.078E−5 1/16 2.555E−6 5.15 9.067E−7 5.08 8.617E−7 6.08 3.035E−7 6.10 1/32 2.267E−8 6.82 6.963E−9 7.02 4.212E−8 4.35 1.088E−8 4.80 表 7 问题3当
$\tau = 0.001,\;t = 0.1$ 时,对不同$ \alpha $ 的$ {L_\infty } $ 误差Table 7.
$ {L_\infty } $ errors for$\tau = 0.001,\;t = 0.1$ with different$ \alpha $ values in problem 3$ \alpha $ $ h = 0.1 $ $h = 0.062\;5$ DHOC[20] present scheme DHOC[20] present scheme $ {10^{ - 1}} $ 4.764E−4 6.692E−4 7.195E−5 1.070E−4 $ {10^{ - 2}} $ 1.264E−4 1.195E−4 3.955E−5 2.632E−5 $ {10^{ - 3}} $ 1.914E−6 1.875E−6 6.366E−7 4.645E−7 $ {10^{ - 4}} $ 1.996E−8 1.963E−8 6.670E−9 4.937E−9 $ {10^{ - 5}} $ 2.004E−10 1.972E−10 6.701E−11 4.967E−11 $ {10^{ - 6}} $ 2.005E−12 1.973E−12 6.704E−13 4.970E−13 表 8 问题3当
$h = 0.062\;5,\;\alpha {\text{ = }}0.1,\;t = 2$ 时,对不同网格比$\lambda = {\tau \mathord{/ {\vphantom {\tau {{h^2}}}} } {{h^2}}}$ 的$ {L_\infty } $ 误差和$ {L_2} $ 误差Table 8.
$ {L_\infty } $ errors and$ {L_2} $ errors for$h = 0.062\;5,\;\alpha {\text{ = }}0.1,\;t = 2$ with different mesh ratio$\lambda = {\tau \mathord{/ {\vphantom {\tau {{h^2}}}} } {{h^2}}}$ values in problem 3$ \lambda $ DHOC[20] present scheme $ {L_\infty } $ $ {L_2} $ $ {L_\infty } $ $ {L_2} $ 1 4.782E−10 1.400E−10 7.213E−10 2.469E−10 2 5.190E−10 1.519E−10 7.231E−10 2.477E−10 4 4.547E−7 1.143E−7 7.255E−10 2.490E−10 6 1.212 2.945E−1 7.468E−10 2.567E−10 8 1.093E+1 2.322 7.326E−10 2.529E−10 -
[1] DOUGLAS J, GUNN J E. A general formulation of alternating direction methods[J]. Numerische Mathematik, 1964, 6(1): 428-453. [2] GUPTA M M, MANOHAR R P, STEPHENSON J W. A single cell high order scheme for the convection-diffusion equation with variable coefficients[J]. International Journal for Numerical Methods in Fluids, 1984, 4(7): 641-651. doi: 10.1002/fld.1650040704 [3] KARAA S. A high-order compact ADI method for solving three-dimensional unsteady convection-diffusion problems[J]. Numerical Methods for Partial Differential Equations, 2006, 22(4): 983-993. doi: 10.1002/num.20134 [4] CAO F J, GE Y B. A high-order compact ADI scheme for the 3D unsteady convection-diffusion equation [C]//2011 International Conference on Computational and Information Sciences. Chengdu, China, 2011: 1087-1090. [5] GE Y B, TIAN Z F, ZHANG J. An exponential high-order compact ADI method for 3D unsteady convection-diffusion problems[J]. Numerical Methods for Partial Differential Equations, 2013, 29(1): 186-205. doi: 10.1002/num.21705 [6] GE Y B, ZHAO F, WEI J Y. A high order compact ADI method for solving the 3D unsteady convection diffusion problems[J]. Applied and Computational Mathematics, 2018, 7(1): 1-10. doi: 10.11648/j.acm.20180701.11 [7] 王涛, 刘铁钢. 求解对流扩散方程的一致四阶紧致格式[J]. 计算数学, 2016, 38(4): 391-404. (WANG Tao, LIU Tiegang. A consistent fourth-order compact scheme for solving convection-diffusion equation[J]. Mathematica Numerica Sinica, 2016, 38(4): 391-404.(in Chinese) doi: 10.12286/jssx.2016.4.391 [8] 罗传胜, 李春光, 董建强, 等. 求解对流扩散方程的一种高精度紧致差分格式[J]. 西南大学学报(自然科学版), 2018, 40(9): 91-95. (LUO Chuansheng, LI Chunguang, DONG Jianqiang, et al. A high-order compact difference scheme for solving convection-diffusion equations[J]. Journal of Southwest University (Natural Science Edition) , 2018, 40(9): 91-95.(in Chinese) [9] SUN H W, LI L Z. A CCD-ADI method for unsteady convection-diffusion equations[J]. Computer Physics Communications, 2014, 185(3): 790-797. doi: 10.1016/j.cpc.2013.11.009 [10] WANG K, WANG H Y. Stability and error estimates of a new high-order compact ADI method for the unsteady 3D convection-diffusion equation[J]. Applied Mathematics and Computation, 2018, 331: 140-159. doi: 10.1016/j.amc.2018.02.053 [11] WU S, PENG B, TIAN Z F. Exponential compact ADI method for a coupled system of convection-diffusion equations arising from the 2D unsteady magnetohydrodynamic (MHD) flows[J]. Applied Numerical Mathematics, 2019, 146: 89-122. doi: 10.1016/j.apnum.2019.07.003 [12] 崔翔鹏, 贺力平. 非线性对流反应扩散方程的预估-校正单调迭代差分方法[J]. 上海交通大学学报, 2007, 41(10): 1731-1736. (CUI Xiangpeng, HE Liping. The prediction-correction monotone finite difference methods for nonlinear transport-diffusion equations[J]. Journal of Shanghai Jiaotong University, 2007, 41(10): 1731-1736.(in Chinese) doi: 10.3321/j.issn:1006-2467.2007.10.037 [13] KARAA S. An accurate LOD scheme for two-dimensional parabolic problems[J]. Applied Mathematics and Computation, 2005, 170(2): 886-894. doi: 10.1016/j.amc.2004.12.031 [14] 赵秉新. 求解一维对流扩散反应方程的高阶紧致格式[J]. 重庆理工大学学报(自然科学), 2012, 26(7): 100-104. (ZHAO Bingxin. A high-order compact difference scheme for solving 1D convection−diffusion−reaction equation[J]. Journal of Chongqing University of Technology (Natural Science) , 2012, 26(7): 100-104.(in Chinese) [15] 杨录峰, 李春光. 一种求解对流扩散反应方程的高精度紧致差分格式[J]. 宁夏大学学报(自然科学版), 2013, 34(6): 101-109. (YANG Lufeng, LI Chunguang. A high order compact finite difference scheme for solving the convection diffusion reaction equations[J]. Journal of Ningxia University (Natural Science Edition) , 2013, 34(6): 101-109.(in Chinese) [16] 杨晓佳, 田芳. 一维非定常对流扩散反应方程的高精度紧致差分格式[J]. 河北大学学报(自然科学版), 2017, 37(1): 5-12. (YANG Xiaojia, TIAN Fang. High order compact difference scheme for the one dimensional unsteady convection diffusion reaction equation[J]. Journal of Hebei University (Natural Science Edition) , 2017, 37(1): 5-12.(in Chinese) [17] KAYA A. Finite difference approximations of multidimensional unsteady convection-diffusion-reaction equations[J]. Journal of Computational Physics, 2015, 285: 331-349. doi: 10.1016/j.jcp.2015.01.024 [18] 张亚刚. 非定常对流扩散反应方程的高精度紧致差分格式[D]. 硕士学位论文. 银川: 宁夏大学, 2018.ZHANG Yagang. High order compact difference scheme for solving unsteady convection diffusion reaction equation[D]. Master Thesis. Yinchuan: Ningxia University, 2018. (in Chinese) [19] LELE S K. Compact finite difference schemes with spectral-like resolution[J]. Journal of Computational Physics, 1992, 103(1): 16-42. doi: 10.1016/0021-9991(92)90324-R [20] YANG X J, GE Y B. A class of compact finite difference schemes for solving the 2D and 3D Burgers’ equations[J]. Mathematics and Computers in Simulation, 2021, 185(2): 510-534. -











 下载:
下载:




























































 渝公网安备50010802005915号
渝公网安备50010802005915号